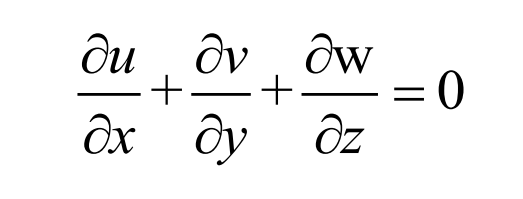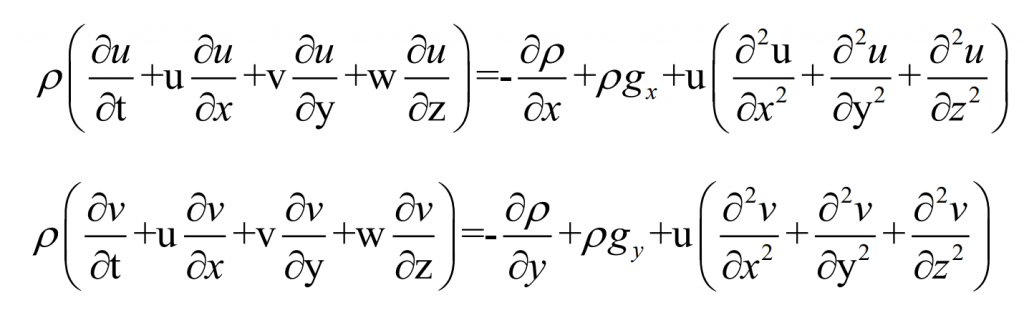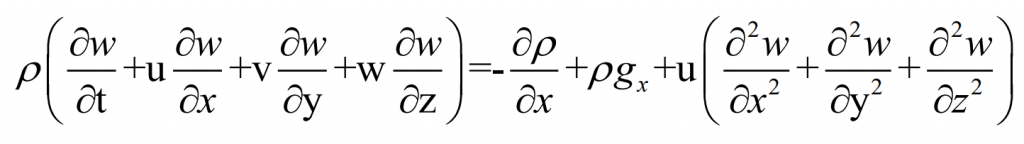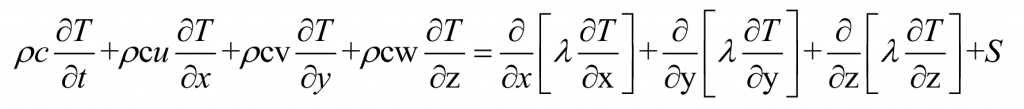In the process of investment casting, although the movement of metal pouring fluid is very complex, it will strictly follow the hydrodynamic laws including momentum conservation, energy conservation and mass conservation, so it can be described by continuity equation, momentum conservation equation and mass conservation equation.
(1) Continuity equation
Because the fluid of investment casting is a continuous medium, the premise of studying the fluid movement is that the fluid continuously fills the occupied space. According to the law of conservation of mass, for a closed surface in a closed and fixed space, when the flow is stable, the inflow mass in a unit is the same as the outflow mass; When the flow is unstable, the mass change in the closed surface is equal to the mass difference of inflow and outflow, which is the continuity equation, and its expression is as follows:
Where u, V and W represent the velocity components of the fluid in the X, y and Z directions respectively.
(2) N-S equation
In the pouring process of investment casting, the fluid is viscous and incompressible, so the momentum conservation equation of the actual fluid can be described by the momentum transfer equation of incompressible viscous fluid, and its mathematical form is as follows:
(3) Energy conservation equation
The law of energy conservation must be satisfied in the filling process of liquid metal in investment casting, which is the most basic and important law, that is, the algebraic sum of internal force work and heat increase in a certain range is equal to the change of energy in that range. The expression is as follows:
Where, C is the specific heat capacity; T is the temperature of alloy liquid; λ Is the thermal conductivity of liquid; S is the internal heat source term; ρ Is the density of the fluid.